Explaining the Sum and Difference of Cosine and Sine

Finding exact values for the tangent of the sum or difference of two angles is a little more complicated but again it is a matter of recognizing the pattern. T F è cos T 18 sin.

Trigonometric Formulas 7 Angle Sum And Difference Identities Segmentation Math Formula

Cos A B cos A cos B sin A sin B.

. C o s L a d j a c e n t h y p o t e n u s e c o s L 12 15. To get the other two product-to sum formulas add the two sine formulas from equation 48 and equation 49 or subtract them. The sum and difference identities of angles are trigonometric identities used to calculate the values of certain angles.

Sine and Cosine of a Sum cosA B i sinA B cos A cos B sin A sin B i sin A cos B cos A sin B So the above equation in sines and cosines is actually two equations one for the real part and one for the imaginary part. You could find the exact value of cos of 15 degrees by using cos45 - 30-cos45 - 30 cos 45cos30 sin45 sin30 1 sqrt2 sqrt32 1sqr. For exact values you will be using opposite signs in the formula.

Finding the sum of two angles formula for tangent involves taking quotient of the sum formulas for sine and cosine and simplifying. Progress Practice Now. T F è sin T REVIEW SKILLZ.

The sum formula for tangent states that the tangent of the sum of two angles equals the sum of the tangents of the angles divided by 1. In summary we have the following two formulas of cosine-sum and cosine-difference. C o s K a d j a c e n t.

Recall tan x sin x cos x cos x 0. No matter the size of the triangle the values of sinθ and cosθ are the same for a given θ as illustrated below. Looking out from a vertex with angle θ sinθ is the ratio of the opposite side to the hypotenuse while cosθ is the ratio of the adjacent side to the hypotenuse.

On the other hand the difference formula for cosines implies that the cosine of the difference of two angles is equivalent to the product of the cosines of the angles adding up the product of the. The cosine of an angle is always the ratio of the adjacent side hypotenuse. The sum formula for cosines states that the cosine of the sum of two angles equals the product of the cosines of the angles minus the product of the sines of the angles.

Cos A B cos A cos B sin A sin B. Sin 2α 2sin αcos α cos 2α cos² α - sin² α where in the cosine formula the exponent applies to the functions value ie cos² α cos α². Cos a cos b - sin a - sin asin b in the angles exact value form then simplify.

The given sine and cosine equation is a combination of functions that fits the difference formula for sine which is sin u - v sin u cos v - cos u sin v. Explain how the sum and difference formula cosA- B cosA cosB - sinA sinB could be useful. Cos a cos b sin a sin b i sin a cos b cos a sin b Equating respective real and imaginary parts cos a b cos a cos b sin a sin b.

So the cosine angle addition formula can be used if you already know the cosine and sine of the. C o s i n e a n g l e adjacent side hypotenuse. The sum and difference formulas for sine and cosine can also be used for inverse trigonometric functions.

Before we finish this section lets apply a nifty trick to turn the above angle sum formulas into angle difference formulas. You will use the sum identity π3 π6. This MATHguide instructional video provides a proof of the sum and difference formulas for sine and cosine.

The sum formula for cosines implies that the cosine of the sum of two angles is equivalent to the product of the cosines of the angles subtracting the product of the sines of the angles. Up to 24 cash back Explain using the sum or difference identities 17 cos. Write each expression as a sine cosine or tangent of a sum or difference of special value angles.

This indicates how strong in your memory this concept is. Review of the Fundamental Trig IdentitiesDeriving the Difference Formula for Cosine from the Unit CircleDeriving the Sum Formula for CosineDeriving the Sum Formula. Find the exact value for sin 12 cos 42 - cos 12 sin 42 using the sine and cosine using the sum and difference formulas.

In this discussion we are going to derive the sum and difference identities also known as the sum and difference formulas for sine and cosine. The difference formula for cosines states that the cosine of the difference of two angles equals the product of the cosines of the angles plus the product of the sines of the angles. Prove the sine and cosine cofunction identities using the sum and difference formulas.

Cos195 cos150 45 cos150cos45-sin150sin45 5. For example to calculate the sine or cosine of 15 we can rewrite 15 as the subtraction of 45 and 30 since the values of the sines and cosines of 45 and 30 are. Cos α β cos α cos β sin α sin β cos alpha beta cos alpha cdot cos beta - sin alpha cdot sin beta cosαβ cosαcosβ sinα sinβ Cosine-difference formula.

½ cos A B cos A B sin A sin B. Applications of Sum and Difference Formulas. Use π3 π4 π6.

Sin105 sin6045 sin60cos45cos60sin45. Sine and cosine aka sinθ and cosθ are functions revealing the shape of a right triangle. The cosine often abbreviated cos is the ratio of the length of the side adjacent to the angle to the length of the hypotenuse.

Cos A B cos A B 2 sin A sin B. 1 2 113 Application and Extension 1 Find the exact value. Tan x sin x cos x cos x 0.

These identities can be used to rewrite the angles as a sum or subtraction of common angles. The sine of one of the angles of a right triangle often abbreviated sin is the ratio of the length of the side of the triangle opposite the angle to the length of the triangles hypotenuse. Sine cosine and tangent sum and difference formulas.

60 45. Here is an outline of this article. You will use sum if angleand difference if angle 90.

Trigonograph Angle Sum And Difference For Sine And Cosine Math Methods Math Visuals Trigonometry

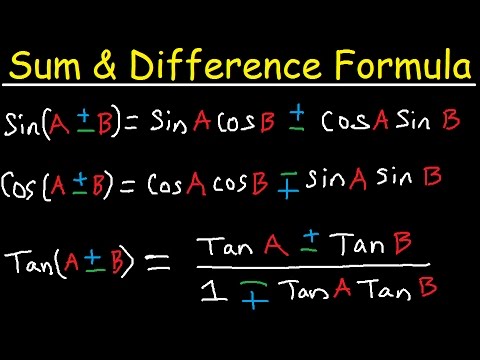
Sum And Difference Identities Formulas Sine Cosine Tangent Degrees Radians Trigonometry Youtube

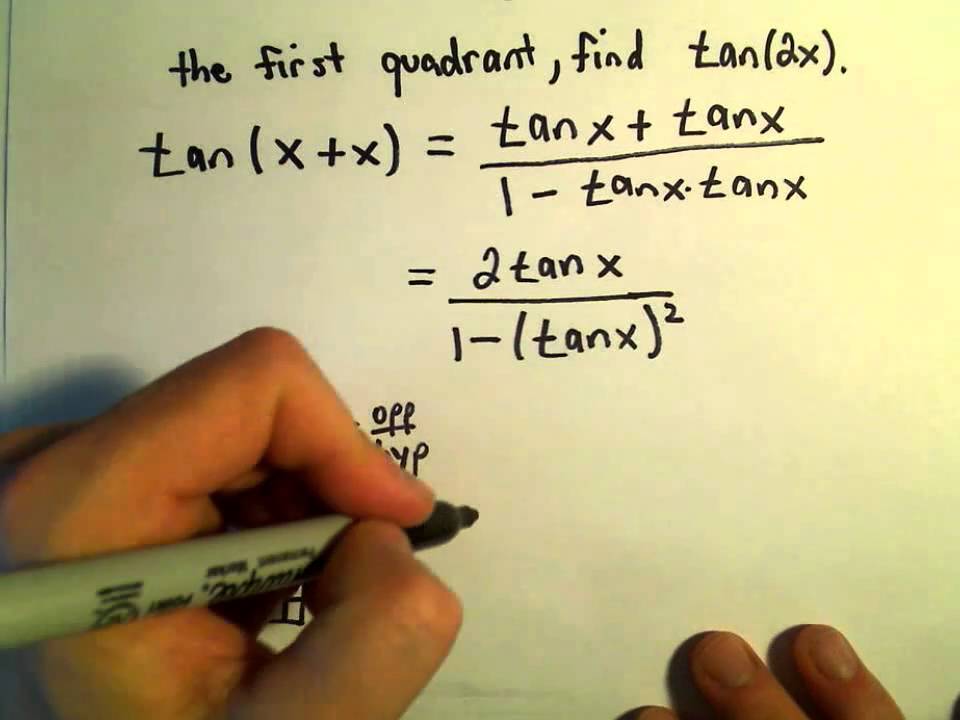
Using The Sum And Difference Identities For Sine Cosine And Tangent Ex 1 Math Lessons Fun Learning Tangent